Solving Quadratic Systems (Jump to: Lecture | Video )

Systems of Equations
Two or more equations put together are called Systems of Equations.

Below, we have a system of equations:

2y = x + 1

3x = 4y - 1

The solution of a system of equations is called an ordered pair (x, y).

Below are examples of some of the linear and quadratic functions we've already learned about:

Figure 1.

Quadratic Systems
Quadratic systems are sets of quadratic equations that have variables with the same values. For example: 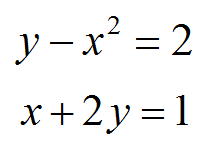The solution of a system of equations is called an ordered pair (x, y). There may be multiple (or no) solutions.

Figure 2.

The above system has a solution at (0, -4).

Figure 3.

The above system has two solutions at roughly (0, -0.2) and (5, 2.8).

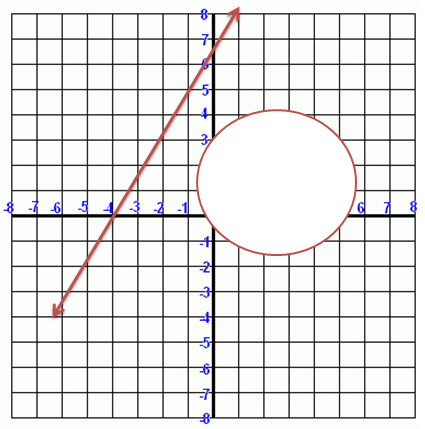
Figure 4.

The above system has no solutions.

Let's solve the system of equations below:

Figure 5.

To graph, first we must convert both equations to slope-intercept form:

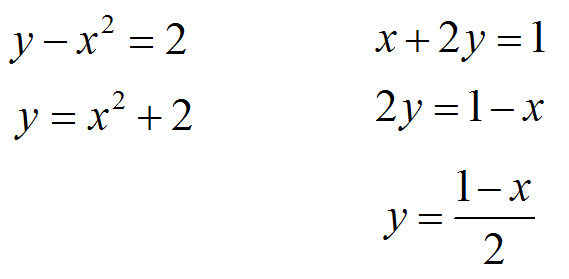
Figure 6.

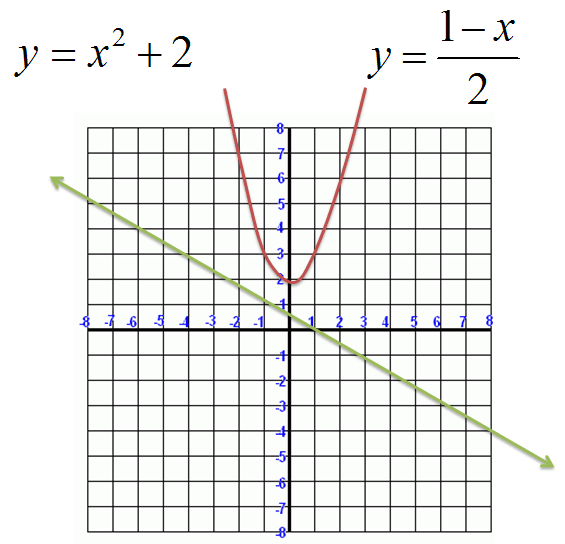
Now, we graph the equations:

Figure 7.

This system of equations has no solution.

---