| Rational Zero Theorem |
|---|
|
If a polynomial equation has integer coefficients, then any rational zero must be in the form of 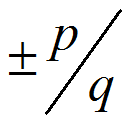
where p is a factor of the constant term, and q is a factor of the leading coefficient. |
Find the rational zeros of:
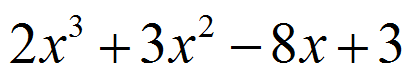
|
Figure 1. |
|---|
First, we determine the factors of the leading coefficient and the constant:

|
Figure 2. |
|---|
Next, we perform synthetic division using each p/q term is the divisor. Any row that has no remainder is a 0 for this function.
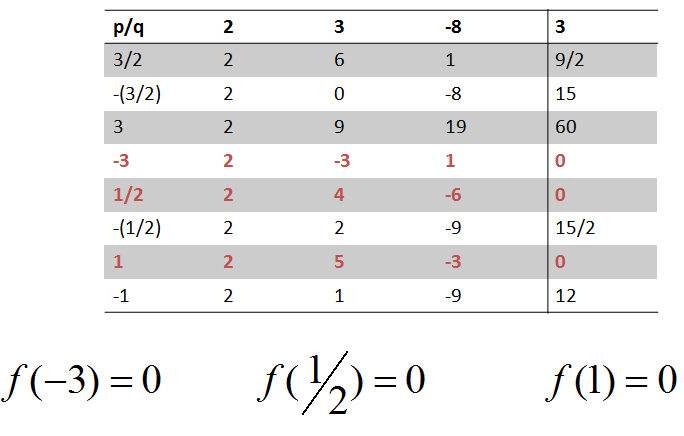
|
Figure 3. |
|---|
The value of the function is 0 when x = -3, x = 1/2, and x = 1.