Imagine a glass that contains 500ml of water. If 12% of the water evaporates every hour, how long will it take for 100ml to remain?
We can solve this problem using the following equation:
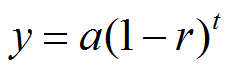
|
Figure 1. |
|---|
y = final amount
a = initial amount
r = rate of decay
t = time
We can arrange our knowns to solve for the unknown, using the logarithmic properties we learned about recently:
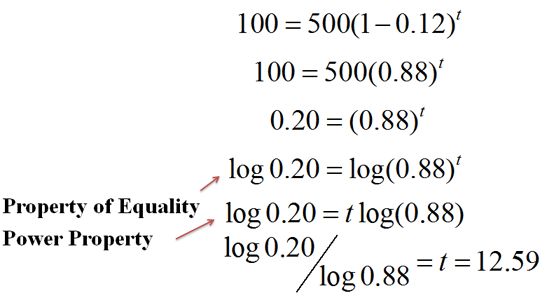
|
Figure 2. |
|---|
It will take 12.59 hours for 100ml of water to remain.
The half-life of a radioactive substance is the time it will take for half of the substance to decay. For example, the half-life for a mass of Carbon is 5730 years.
We can solve this problem using the following equation:
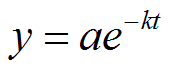
|
Figure 3. |
|---|
What is k? That is, what is the rate of decay of Carbon?
y = final amount
a = initial amount
e = natural base
t = time
Again, we can arrange our knowns to solve for the unknown, using the logarithmic properties we learned about recently:
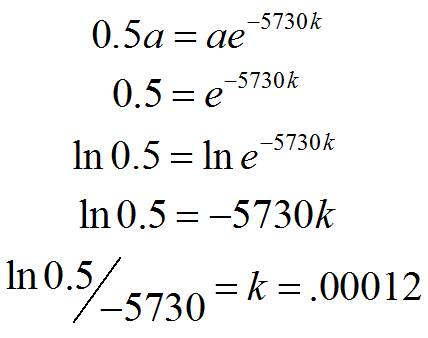
|
Figure 4. |
|---|
The rate of decay of Carbon is k = .00012